Technical Papers and Presentations

The low dimensional systems show a behavior that is strongly depending on external effects such as the intense non-resonant laser field. The control of electronic and optical properties by means of a laser field lets us to adapt the matter-light interaction to several applications [1].

In this work, it is used the Coefficient Form PDE interface of the COMSOL Multiphysics^® simulation software to calculate the energies, wavefunctions, and dipolar matrix elements of an electron confined in a GaAs quantum ring (QR) and embedded in a matrix of AlGaAs. These amounts are calculated for both with and without laser-field effect presence.

In order to implement the model in COMSOL Multiphysics^®, the following procedure was carried out:

• The physical parameters of the model were defined

• The quantum ring geometry was built with two ellipses

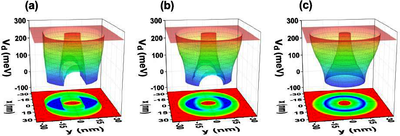
• Due to the inside and outside of the QR for the potential, the effective mass, and the laser-parameter take different values, Heaviside step functions were used to define these three parameters depending on the position

• In order to fulfill the normalization criterion for the wavefunctions, it was necessary to define a new variable by using integration on the geometry of the non-normalized wavefunctions

• The laser-dressed confinement potential is defined as a variable, which is obtained by integrating the potential on a period. In terms of the Finite Element Method, this means that we integrate the dressed potential on a period in every nodal point

• The Schrödinger equation is defined in two coefficient forms PDE depending on the region. This is, the inner and outer of the QR take different coefficients as a function of the material parameters.

• A variable to calculate the matrix dipole elements is built, by using the previously defined normalized wavefunctions and taking a linear or circular polarization

• The results for energies, wavefunctions, and dipolar matrix elements with and without laser field effect are taken from the derived values

Additionally, parametric sweeps were implemented, which is useful to observe parameter change effects such as the dimensions and the laser-parameter (related to the laser field intensity).

[1] V.M. Fomin, Physics of Quantum Rings, second ed., Springer, Berlin, 2018.