Technical Papers and Presentations

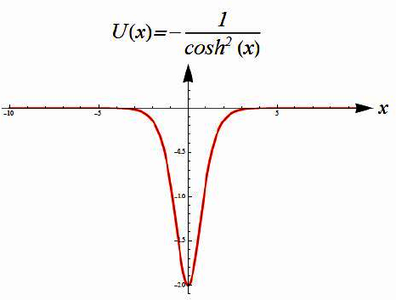
This talk communicates a pseudo-dynamic approach to solve nonlinear stationary equations exhibiting soft bifurcations. The procedure consists in passing from the stationary partial differential equation - to an artificially-built pseudo-time dependent one. We construct the latter in such a way that the desired nontrivial solution of the stationary equation represents its fixed point. The numeric solution of the stationary equation which is being looked for is then obtained as the solution of the pseudo-time dependent equation at a high enough value of the pseudo-time. The approach application is demonstrated within an example of a 1D inhomogeneous Ginzburg-Landau equation.