Mode analysis is an essential tool in radiofrequency and wave optics calculations because it allows you to investigate mode characteristics in complex waveguide structures. In this blog post, I will provide an introduction to mode analysis and will summarize the physics interfaces, study steps, and postprocessing settings you will need to carry out this type of study in the COMSOL Multiphysics® software. Then, I will show several examples of pure mode analysis. Lastly, I will explain how to use the results for further calculations of complex RF and optical waveguide systems.
Table of Contents
- What Is Mode Analysis?
- How to Set Up Your Model for Mode Analysis
- Tutorial Model Examples of Mode Analysis
- Excitation or Termination of Propagating Modes in Frequency-Domain Waveguide Calculations
- Conclusion
What Is Mode Analysis?
When analyzing an arbitrary 3D waveguide structure, it is important to understand which types of electromagnetic waves are allowed to propagate at a given frequency. These wave regimes are determined by the resonant modes, which can be excited in a 2D transverse cross section of a waveguide. Such modes can be fully described by the global complex-valued propagation constants and the space distributions of all three components of electric field (so-called mode shapes). Transmission regimes in waveguides with a constant cross section can be defined completely based on these electromagnetic characteristics. We can also use this information for frequency-domain studies of scattering characteristics in more complex structures.
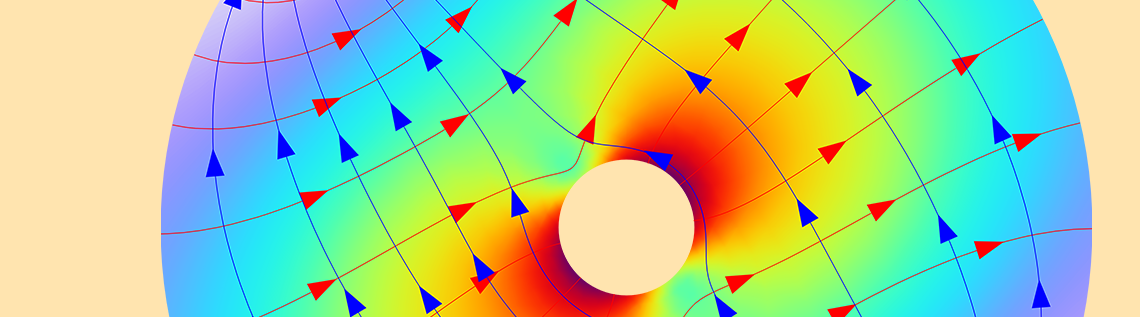
Well-known analytic solutions are only available in the literature for some RF designs, such as coaxial lines and hollow waveguides with rectangular or circular cross sections. For any other configuration with arbitrary shape and material combinations — including all typical optical fibers and integrated waveguides — it is necessary to use numeric mode analysis. Figure 1 shows the formulation of numeric mode analysis in the Equation section of the Settings window. To perform a mode analysis, you will need to plug a given frequency into the Helmholtz equation for electric fields and then search for a solution in the form of a wave traveling in the out-of-plane direction. For that purpose, you can use the finite element method (FEM) and an eigenvalue solver.
Note: Mode analysis should not be confused with the more general modal analysis. The latter is referred to as eigenfrequency analysis and can be used for finding resonant or natural modes and eigenfrequencies in a system of any possible dimension, including 2D, 2D axisymmetric, and 3D.
You can perform mode analysis in the RF Module or Wave Optics Module, both add-on products to COMSOL Multiphysics, by using the following features: the Electromagnetic Waves, Frequency Domain physics interface for 2D or 2D axisymmetric geometry and a Mode Analysis study.

Figure 1. Mode analysis for an optical waveguide in COMSOL®. The formulation of such a study is shown in the Equation section of the Settings window for the Electromagnetic Waves, Frequency Domain physics interface.
How to Set Up Your Model for Mode Analysis
Geometry and Material Settings
When setting up a model for mode analysis, you should first build transverse cross sections of a waveguide structure. You can make it directly in 2D or you can reduce a 3D model using the Cross Section operation.
Then, you can specify materials properties and assign them to the corresponding parts of the geometry. For radiofrequency models, you will usually need electrical conductivity, relative permittivity, and relative permeability. For wave optics models, you will usually need a refractive index. COMSOL® automatically converts material data from one representation to another.
Using nonzero conductivity, complex-valued relative permittivity, and complex-valued refractive index will introduce damping in the model, which you can observe in postprocessing.
Physics Settings
The goal is to find a wave that is propagating in the out-of-plane direction. To do so using the Electromagnetic Waves, Frequency Domain physics interface in 2D, open the Settings window for the physics interface and make sure the Three-component vector option is selected in the Component section.
Mode analysis is an eigenvalue study, so there is no need to use any source conditions. However, you should still define proper boundary conditions since they will have an affect on mode shape and mode damping and leakage. Note that external boundaries can be metallized or open. If you are working with metallized boundaries, you can use the default Perfect Electric Conductor or Impedance Boundary Condition. In order to describe open boundaries, you can use the Scattering Boundary Condition or a Perfectly Matched Layer.
The default settings for the Scattering Boundary Condition and a Perfectly Matched Layer work well in situations where the electromagnetic wave moves in the normal direction toward the boundary. Such default settings are not optimal for mode analysis since the wave vector of interest is composed of the propagation constant, which is directed tangentially to the boundary, and a remaining normal component. You should manually tweak the settings for the effective wavelength in the Perfectly Matched Layer feature or enable the Subtract propagation constant from material wave number check box in the Mode Analysis section of the Settings window for the Scattering Boundary Condition. You can find detailed instructions on how to do so in the Leaky Modes in a Microstructured Optical Fiber tutorial model’s PDF documentation.

Figure 2. Mode analysis for a coaxial cable in COMSOL®. Using the Impedance Boundary Condition allows you to calculate both propagation and attenuation constants.
Using the Impedance Boundary Condition, Scattering Boundary Condition or Perfectly Matched Layer feature will introduce damping into the model.
Mesh and Study Settings
A variant of the Mode Analysis study settings for electromagnetics problems is shown in Figure 3 below. By default, the Effective mode index transform is selected, which is usually the best choice for electromagnetic waves. With such a transform, you can assume that the so-called effective index of mode (or effective refractive index) will be used as a declarative characteristic of the mode.
In the Mode analysis frequency field, you should enter the frequency at which you want to find resonant modes. As you can see below, the next study setting listed is Mode search method. If Manual search is selected here, you should set the initial guess in terms of the effective index of mode in the Search for modes around field and the Desired number of modes. The solver will search for modes near that guess and return the expected number of different modes if possible. For the Region search, you should specify the approximate number of modes and the region of the complex effective index.
You might wonder how to make a good initial guess for a successful calculation. It’s actually case-specific, but I have a basis recommendation for you if you work with typical waveguide structures that consist of core and cladding: In this situation, the modes of interest have effective mode indices somewhere between the refractive coefficients of the two materials, and the fundamental mode has the highest index. Therefore, setting the initial guess value to be near the core refractive index in the Search for modes around field guarantees that the solver will find the fundamental mode. In other words, the effective index for the fundamental mode of a typical waveguide structure is close to the refractive index for the domains where the mode energy will be confined.
You can add a Parametric Sweep, select any predefined parameter — such as the geometric size, material property, or frequency — and change its value through a specified range. As a result, you will get what are known as dispersion curves. You can even define cut-off conditions for different modes.

Figure 3. Settings for the Modal Analysis and Parametric Sweep study steps. You can see in the expanded Solver Configurations section that an Eigenvalue Solver is used for the study.
While we skipped the mesh settings and chose the study settings first here, note that the entered frequency value in the Mode analysis frequency field is also used for Physics-controlled mesh generation. By default, the software uses five elements per wavelength for each material. For better resolution, you can refine it. For 2D models, such refinement won’t cause any notable memory consumptions.
Postprocessing and Results Interpretation
Let’s discuss the typical results that you can get after running the Mode Analysis study.
For each calculated mode, you can plot local field or power flow distributions. You can choose the x-, y-, or z-component or the norm value as an expression. This will allow you to easily define the field profile and polarization. You also have several global variables based on eigenvalue lambda and complex propagation constant alpha=-lambda for each mode, including the propagation constant, attenuation constant, and effective mode index. You can obtain their exact values via the Global Evaluation feature or using 1D Global plots. The names and definitions of the available variables are summarized in the table below:
| Name | Expression | Description | Units |
|---|---|---|---|
beta |
imag(alpha) |
Propagation constant | rad/m |
dampz |
real(alpha) |
Attenuation constant | rad/m |
dampzdB |
20*log10-(exp(1))*dampz |
Attenuation in logarithmic scale | dB/m |
neff |
-j*alpha/k0 |
Effective mode index | 1 |
Note: In 2D axisymmetry, an extra normalization is included for the complex propagation constant,
alpha=-lambda/Rave, whereRaveis the average radius of curvature for the created geometry. If necessary, a user can define another value forRavewithin the Average Radius section of the root interface node.
For TEM (or quasi-TEM) modes, you can manually calculate the characteristic impedance via the integrals for the current and voltage. The details are discussed in a previous blog post about the usage of numeric ports in RF analyses.
Usually, postprocessing of mode analysis is straightforward, but questions about the correct interpretation of results often come up for the following cases:
- Mode analysis is an eigenvalue study, so exact field amplitude is rather arbitrary and only the relative proportion matters. In postprocessing, you can perform additional normalization for each mode.
- If eigenvalues of two different modes are very close to each other, then you will get any linear combination of them after calculation.
- Calculated modes are just sets of orthogonal solutions that are sorted by the effective mode index. Since the software can’t detect exact physical types of modes, it doesn’t “know”, for example, that the first mode is TE11 and that the sixth mode is TM20. This means that you need to identify the physical type of mode yourself via a manual analysis of field distributions.
- The sorting of modes is performed for each parameter value in a Parametric Sweep, so the order of modes can be swapped while moving from one parameter to another. For this, it’s better to plot raw dispersion curves as a set of points rather than a set of lines. To get line representation afterward, a kind of manual sorting is required. As an alternative to the hand sorting, one can try introducing a kind of mode tracking in postprocessing. For instance, one can evaluate the mode overlap integral or compare the originalities of modes for close parameter values.
- Some modes can be unphysical, but you can identify them based on their field profile. In these cases, distributions can be very jagged or can have maxima near the external boundaries.
- Sometimes, modes have a very small propagation constant (close to zero) and a small effective index. You can define them as nonpropagating or evanescent. If you perform a sweep via a relevant control parameter, e.g., frequency, then you can track the cut-off conditions for such a mode.
- Effective mode index can be complex-valued. You can obtain a large imaginary part in two main situations: if the mode is evanescent or if there is some source of damping in the system. Damping can be introduced by a material property, metallization with finite conductivity, and/or an open boundary.
Tutorial Model Examples of Mode Analysis
Let’s walk through some models that demonstrate two different ways to perform mode analysis in COMSOL®, with extended settings and postprocessing. Below, you can find more information about their setup.
If you would like to jump right into building these models yourself, you can download them here: “Mode Analysis for Electromagnetic Waveguides”.
Example 1: Mode Analysis for a Coaxial Cable
Let’s start with an RF example and calculate the modes of a typical coaxial cable. A cable under observation has an inner radius, r_i = 0.5 mm; outer radius, r_o = 3.43 mm; insulator relative permittivity, eps_r = 2.4; and conductor parts made from copper. Our goal is to define the characteristics of the main TEM-mode and first higher-order TE11-mode in a range of frequencies from 10 to 20 GHz.
Setting up the model is very simple. First, we define the geometry without metal parts. Then, we add the generic Insulator material for a 2D domain. Next, in the Electromagnetic Waves, Frequency Domain interface, we set the Impedance Boundary Condition for external boundaries and assign the build-in Copper material to them. In the Mode Analysis study step, our settings are f0 for Mode analysis frequency, 2 for Desired number of modes, and sqrt(eps_r) for Search for modes around. We also add a Parametric Sweep with f0 as the parameter and enter our frequency range with a small step of 0.2 MHz.
Mode analysis provides two solutions for each frequency. We can examine them via space distributions and global variables. From the two solutions, the one that has an effective index closest to the value of sqrt(eps_r) ≈ 1.55 is the one that is the TEM-mode. It has a monotonically growing propagation constant and a small attenuation, below 1 dB/m, in our frequency range. Another solution with a smaller index is the TE11-mode. Both its effective index and propagation constant are very small at the beginning of the frequency range; the attenuation constant is very high in contrast. All of these factors indicate that this mode is nonpropagating for the beginning of the frequency range.
Figure 4. The space distributions of several modes for a coaxial cable. Here, we can see the TEM-mode at 10 GHz (upper-left) and at 20 GHz (upper-right) as well as the TE11-mode at 10 GHz (lower-left) and at 20 GHz (lower-right). The surface plot is for the Z-component of power flow, the red streamlines are for the electric field, and the blue are for the magnetic field. Annotations are used for highlighting propagation and attenuation constants. Note that the TE11-mode for 10 GHz has a very small effective index that is an indicator of an evanescent regime.
To define the cut-off frequency, we can create global 1D plots of emw.beta and emw.dampzdB and use f0 as the expression for x-Axis Data. The sharp jump of the TE11 curve that happens around 15.6 GHz is a good estimation of the cut-off condition.
Figure 5. Propagation constant and attenuation constant as functions of frequency for the TEM-mode and the TE11-mode.
For TEM-mode, we can also evaluate characteristic impedance. Values of the characteristic impedance and cut-off frequency for TE11-mode are good verification factors for numeric mode analysis in COMSOL® because we can compare them with well-known analytic expressions.
Example 2: Mode Analysis for a Rib Waveguide
Let’s continue with a wave optics example and calculate the modes of a typical integrated waveguide. The rib waveguide under observation has a core made from Silicon, an upper cladding made from SiON, and a lower cladding made from SiO2. The refractive indices of these materials are n_core = 3.48, n_clad_upper = 1.51, and n_clad_lower = 1.44, respectively. Our goal is to define all possible modes at a certain wavelength, lda0 = 1.55 um; for the fixed core height, h_core = 700 nm; and for various values of the core width, w_core.
Like the previous example, setting up the model for this case is also straightforward. First, we define a geometry that contains three domains for the core, the upper cladding, and the lower cladding. Then, we assign them to materials with optic properties (as described in the previous paragraph). Next, in the Electromagnetic Waves, Frequency Domain interface, we set Scattering Boundary Condition for external boundaries and enable the Subtract propagation constant from material wave number check box. In the Mode Analysis study step, our settings are f0= c_const/lda0 for Mode analysis frequency; 10 for Desired number of modes; and n_core for Search for modes around. We also add a Parametric Sweep with w_core as the parameter and vary it from 300 nm to 1000 nm.
Mode analysis returns ten solutions for each frequency, which we can examine via space distributions and global variables. We can use arrows or streamlines to visualize polarization for each mode and define its type, – EyMN or ExMN. The superscript denotes the dominant polarization direction and the first and second subscript variables denote the number of peaks in the x and y direction, respectively. We can also observe high-order modes, like Ey22 and Ey31, for large values of the w_core parameter.
Figure 6. Space distributions of several modes for a rib waveguide. In the upper row we can see the Ey11-mode for a core width of 400 nm (left) and the Ex11-mode for a core width of 400 nm (right), while in the lower row we can see the Ex11-mode for a core width of 1000 nm (left) and the Ey21-mode for a core width of 1000 nm (right). The surface plot is for the Z-component of power flow, and the black arrows represent the electric field. Annotations are used for highlighting the effective mode index and propagation constant.
We can also use the global 1D plot of ewfd.neff with w_core*ewfd.k0 as the expression for x-Axis Data to obtain a typical dispersion curve. Using these plots, we can track very complex behavior in a rib waveguide. We can define the exact points where “swaps” of different modes take place; e.g., Ey11 and Ex11 (around w_core*ewfd.k0 = 2), Ey21 and Ex21 (around w_core*ewfd.k0 = 3.5), etc.
Figure 7. Dispersion curves for a rib waveguide shown in a point plot (left) and line plot (right) after manual mode tracking based on the move overlap integral. Many mode “swaps” are visible, e.g., there are shifts of Ey11 and Ex11 around w_core*ewfd.k0 = 2 and of Ey21 and Ex21 around w_core*ewfd.k0 = 3.5.
Note that for a small value of core width, we also obtain several modes with an effective index below the refractive index of cladding. Their spatial distribution shows that energy does not confine inside and around the core. We can ignore such modes as unphysical and filter out in postprocessing or even remove them via the Remove solution study feature.
Other Available Examples
Apart from the two models presented above, I recommend exploring the following examples from the Application Gallery:
- Step-Index Fiber Bend
- Slot Waveguide
- Optically Anisotropic Waveguide
- Stress-Optical Effects in a Photonic Waveguide
Excitation or Termination of Propagating Modes in Frequency-Domain Waveguide Calculations
Once you have found propagating modes, you may want to excite or terminate one or several of them in a waveguide cross section during a frequency-domain analysis. This will allow you to calculate the reflection and transmission characteristics in a complex electromagnetic device. The capabilities of the RF Module or Wave Optics Module allow you to perform these operations.
For these types of modeling scenarios you can use the Electromagnetic Waves, Frequency Domain or Electromagnetic Waves, Beam Envelopes physics interface. Then, you would typically add the Port boundary condition on each relevant cross section for each relevant mode. After that, you would enable the Numeric option for each Port. For simplicity, let’s call the Port feature with such modifications Numeric Port. For calculation, you could use a combination of the Boundary Mode Analysis for each Numeric Port and Frequency Domain study.
Using a Numeric Port implies that the mode profile and its propagation constant will be calculated during the Boundary Mode Analysis study step, which is almost identical to Mode Analysis. There is one additional Port Name setting where you should specify an exact port name, and the study will perform mode analysis for all the boundaries of this Port. Note that you need to find one exact mode for each port, so you should set the Desired number of modes to 1 and enter a very accurate estimation for the effective mode index in the Search for modes around field. If you do not have any prior knowledge of mode characteristics, it would be a good idea to perform a preliminary mode analysis and define global values for all needed modes — this is especially important when working with the multimode regime. It is also possible to automate a subsequent setup of ports.
This setup is very powerful. First, you can use it directly in 3D without creating a 2D cross section and, moreover, you can use it in 2D for 1D ports. Second, in postprocessing, you receive S-parameters and the reflection and transmission coefficients for a device. Third, you can define a typical wavelength for a Perfectly Matched Layer or specify a phase in the Wave Vector settings of the Electromagnetic Waves, Beam Envelopes physics interface using a propagation constant directly from Boundary Mode Analysis.
Note: For RF devices like microstrip or coplanar lines, there are some special settings for Numeric Port in order to receive TEM or quasi-TEM mode.

Figure 8. The frequency domain analysis of the optic waveguide with a scatterer. Four Numeric Ports are used in this model. You can see the Settings window of the Boundary Mode Analysis for the second Numeric Port, which is used to terminate fundamental mode. Information about its effective index is obtained via a preliminary study.
There are several great examples in the Application Gallery that showcase the use of the Numeric Port conditions and Boundary Mode Analysis studies, such as the:
- Optical Ring Resonator Notch Filter model
- Directional Coupler model
- RF Waveguide Adapter model
- Mach–Zehnder Modulator model
- Tapered Waveguide
Conclusion
In this blog post, we went over how to use the RF Module or Wave Optics Module to find resonant modes in cross sections of waveguide structures and get their qualitative and quantitative characteristics, which can be used in further full-wave studies for the excitation or termination of such modes. In addition, we looked over the typical settings of models with the Mode Analysis study and Numeric Ports. With this information, we can improve the efficiency of our radiofrequency and wave optics calculations.
The techniques discussed here can also be used to investigate wave behavior in acoustics and mechanics applications. For more details, please check out the following models: mode analysis of the muffler cross section with rigid and elastic walls, investigation of elastic wave propagation in a plate, and aeroacoustic calculations of the aero-engine duct.
Next Step
Try performing mode analysis for a model of a coaxial cable or a rib waveguide yourself using the Mode Analysis for Electromagnetic Waveguides tutorial model:
Editor’s note: This blog post was updated on October 16, 2024 to include more information on results interpretation.




Comments (47)
Cory Cress
July 19, 2022Can you explain why you are using w_core*ewfd.k0 as the x-axis to plot the dispersion?
Sergey Yankin
July 25, 2022 COMSOL EmployeeHi, Cory!
In this case it’s a kind of normalization (in fact w.r.t. wavelength) which is quite useful and popular in optics literature. In your own model you can use any arbitrary expression for x-Axis Data.
Asma Mimouni
November 9, 2022Hello,
Thank you for the detailed explanations.
Is it possible to perform a mode analysis of an optical fiber in cylindrical coordinates?
Thank you
Sergey Yankin
November 9, 2022 COMSOL EmployeeHi, Asma!
Mode analysis is performed in Cartesian coordinates by default. But you can represent your results in cylindrical manner if needed in postprocessing
Daniel Jedrzejczyk
March 29, 2023Dear Sergey,
thank you for a very interesting blog post. Is there any particular reason why the fundamental modes in the examples above are labeled with subscript indices of 1? Is this a matter of nomenclature? IMHO these should be labeled with 0 similar to transverse modes of laser beams. In this case the index number corresponds to the number of roots in the transverse electric field distribution.
Kind regards,
Daniel
Sergey Yankin
April 6, 2023 COMSOL EmployeeHi Daniel!
Are you talking about TE11/Ey21/Ex21 labels? If so, it’s just a common convention for this scientific area.
Ramin
July 13, 2023Hi,
according to you, the mode analysis is performed in Cartesian coordinates by default and we can represent the results in cylindrical coordinates in post-processing. So, could you please tell me, how I can do that? thanks in advance.
Sergey Yankin
July 18, 2023 COMSOL EmployeeHi Ramin!
As one variant you could define additional Coordinate System & Vector Transform in the Definitions node and use these supplementary variables during postprocessing. For details please refer to the following COMSOL tutorial: https://www.comsol.com/model/static-field-modeling-of-a-halbach-rotor-14369
Agraj Yadav
August 28, 2023Hello sir,
I am doing mode analysis in Liquid crystal-based long-period waveguide grating in step-index silicon on insulator(SOI), In which there is a silicon core of refractive index 3.4506, liquid crystal cladding of refractive index 1.5, and Silica(SIO2) with refractive index 1.446 .so here I want to extract the data of effective mode indices of the fundamental mode and higher order mode with different Wavelengths ranging from 1.46e-6 m to 1.62e-6 m with a step size of 0.0025e-6 m. one thing is that how can I Recognise a particular higher order mode like TE01 or TE10 or something like that so that I able to extract data of fundamental mode field profile and a particular higher-order slab mode field profile using Comsol with Matlab Simulink. So please kindly resolve my problem.
Sergey Yankin
August 28, 2023 COMSOL EmployeeHi Agraj!
As I’ve already stated in the article, there is no automated way to identify the physical type of mode right after the mode analysis study. So, you have to perform such a recognition manually or via some user-defined case-specific macros/script.
Naftali Herscovici
September 19, 2023Hi Sergey,
Is it possible to get the list of the cutoff frequencies for all the modes computed?
Thank you
Sergey Yankin
October 4, 2023 COMSOL EmployeeHi, Naftali!
If you just need a list of cutoff frequencies, then you might want to use the Eigenfrequency study instead of the Mode Analysis setup.
Wu XianShi
October 7, 2023Hello Sergey,
Thanks for your blog. I’m now trying to analyze the fiber eiginmodes and their losses in a 2D transverse cross section using Mode Analysis with Scattering Boundary Condition. Should I enable the Subtract propagation constant from material wave number check box in the Mode Analysis section of the Settings window for the Scattering Boundary Condition? And should I choose Cylindrical wave from the Scattered wave type list instead of Plane wave (the default)? In the Leaky Modes in a Microstructured Optical Fiber tutorial model’s PDF documentation it did both, but I really don’t know why. Can you tell me the right settings and the reasons?
Thanks.
Sergey Yankin
October 10, 2023 COMSOL EmployeeHello!
The Scattering Boundary Condition is meant for open boundary handling and is useful if you need to investigate various leaky modes. Is it in line with your case? If so, you do need that checkbox. Please check the comprehensive explanation here (p.7 of the PDF-instruction): https://www.comsol.com/model/leaky-modes-in-a-microstructured-optical-fiber-88741
The Scattered wave type of the Scattering Boundary Condition just should be consistent with the wave type that you expect to propagate towards selected boundaries.
Alan Lu
January 3, 2024Hi Sergey,
Thanks for the detailed explanation and tips which is very helpful for me.
Just curious what if the optical waveguide is not rib one, but a classical slab waveguide that has infinite length on its x direction? I know there is a sample model for the slab waveguide provided from Comsol, but it is using the port mode analysis without the need to consider the infinite dimension.
Regards,
Alan
Sergey Yankin
January 10, 2024 COMSOL EmployeeHi Alan!
I’m not sure that I follow your question well. From general considerations, the Boundary Mode Analysis in 2D should handle this task. If it’s not the case, please submit a support request and our team will assist you!
Sankar Ganesh Ramaraj
March 11, 2024Dear Sergey san,
Thank you for explaining. I need some explanation with my experiments
John
May 1, 2024Hi Sergey, useful blog post. I’m looking to extend the mode application. Consider a 3D RF problem with which is uniform in z for z 0 (but different, like interface between two microstrip). Assume excitation in the lower field. The field for z 0. COMSOL offers Frequency Domain Modal which would find some suitable combination of 3D eigenmodes over the entire domain. Alternatively in each subdomain, I can take modes on cross-section. But what I’m finding tricky is a way to glue the ideas together. In the lower field I should be able to write the field as sum of lower 2D modes weighted as exp(ik |z – z0|) + R exp(-i k z) and in the upper field I should be able to write the field as sum of the upper modes weighted by T exp(i kt z). No 3D eigenfunctions required, just projections of the 2D modes. The solution would be given as a small matrix inversion where each component would be a cross of one 2D eigenmode against another – much as the Modal method matches boundary conditions now. Does COMSOL have an example like that? Would make for a great blog entry… 🙂
Sergey Yankin
May 20, 2024 COMSOL EmployeeHi John! I’m not sure that I follow your question well and I’m afraid you’re mixing the definitions of the Mode Analysis and Eigenfrequency Analysis which are a bit independent. If the question is still relevant, please submit a support case with a test model, so you can discuss it in detail with my colleagues or with me.
Lisa Haerteis
July 3, 2024Hi Sergey,
thank you for this detailed description. It is very helpful. I have a few qestions:
1) how can I find out about all the available variables, just like ewfd.neff etc etc? Is there a list somewhere?
2) how do you create the dispersion curve in Fig 7? I started my own simulation where I solved for 10-10 modes and their respective effective mode indices for different waveguide widths. However, I can’t figure out how to create this dispersion diagram in COMSOL.
Thank you!
Lisa
Sergey Yankin
July 4, 2024 COMSOL EmployeeHello Lisa!
1. The full list of variables usually can be checked via the so-called Equation View regime: https://www.comsol.com/support/learning-center/article/Viewing-and-Accessing-the-Equations-and-Variables-for-Physics-Feature-Nodes-30761/122
2. The model with that plot is available for download. If you have problems adapting those settings to your own project, I recommend submitting your question with a model itself to our support portal. We’ll be happy to help you!
Best regards
Sergey Yankin
Kolok Baksa
July 8, 2024Hi Sergey,
I am studying the vibrations of a rod with Mode Analysis. In this case, there are multiple solutions for different frequancies. If I make a parametric sweep for the frequency parameter I found the dispersion curves. Do you know any efficient ethod how to separate the dispersion curves for the different modes? My solution would be a comparison of the solutions corresponding to different frequencies, like calculating an overlap between the polarization vectors, to find a correspondence between the modes with different frequencies. However, I could not find the way to do this in COMSOL.
Sergey Yankin
July 8, 2024 COMSOL EmployeeHi Kolok! Please check out the following suggestion by my colleague: https://www.comsol.com/forum/thread/240122/eigenfrequencies-sorted-by-eigen-modes
Kolok Baksa
July 9, 2024Thank you for the quick answer. The link you have attached is very useful!
Liu Yiwen
July 26, 2024Hi Sergey,
Thanks for the nice tutorial.
I’m working on a periodic grating waveguide and using the continuity periodic condition. I tried performing mode analysis to obtain the dispersion curves, but the correct modes cannot be found. However, these modes can be identified using eigenfrequency analysis.
Is mode analysis unsuitable for periodic waveguides, or is there an error in my settings?
Thanks,
Yiwen
Sergey Yankin
July 26, 2024 COMSOL EmployeeThe Mode Analysis is meant for a cross-section of a waveguiding structure, so I’m unsure how you’re going to apply it for a periodic grating waveguide. Can you elaborate on that a bit? I would recommend you submit a support request via your COMSOL Access account.
Liu Yiwen
July 26, 2024Hi Sergey,
I only drew one unit cell in the geometry, similar to a ridge waveguide. For the periodicity, I set the two vertical edges with periodic conditions and the two horizontal edges with scattering conditions.
Sergey Yankin
July 26, 2024 COMSOL EmployeeThe Mode Analysis assumes that the sought mode propagates in the out-of-plane direction. I suspect this is not in line with your design and idea.
Liu Yiwen
July 26, 2024Thanks.
I understand that Mode Analysis assumes the mode propagates in the out-of-plane direction, which may not align with my design for the periodic grating waveguide, where the mode propagates along the plane.
What specific analysis tools or methods would you recommend for accurately analyzing modes in a periodic waveguide?
Sergey Yankin
July 26, 2024 COMSOL EmployeeI would recommend you submit a support request via your COMSOL Access account so that we can discuss it in detail
Дмитрий Жабровец
October 5, 2024Thank you for the article. Please answer my question. When I model a waveguide in the longitudinal direction, I manage to get a fundamental mode, but it is polarized only in one direction (in z), although it should be polarized in z and y. In the listed examples, as well as other examples on the site, everywhere during modeling the light is polarized only in the z direction, although when modeling a waveguide in the transverse direction it is possible to find both TE and TM modes
Sergey Yankin
October 8, 2024 COMSOL EmployeeHello!
First, it’s hard to say anything definite without checking your model itself and knowing the full problem statement. That said, please submit a support request so that we can discuss it closely.
Second, I’m not sure I follow your second statement regarding COMSOL’s tutorials and mode polarization there. For instance, in the second model showcased in this blog post, the fundamental mode has the Y-polarization…
Дмитрий Жабровец
October 8, 2024Thanks for the answer.
Sorry for writing unclearly.
The second example in the blog is an example of a waveguide in a cross-section. It is easy to get modes with different polarizations there.
I mean the case when the length of the waveguide (fiber) is important to us, when we model the field in the longitudinal direction through the ports, as for example in the model in Figure 8 (waveguide_with_scatterer) (in this model, the researchers also set the polarization only in the z direction)
Sergey Yankin
October 11, 2024 COMSOL EmployeeDear Dmitry!
First of all, Mode Analysis (MA) in 2D and Boudary Mode Analysis (BMA) in 3D are conceptually the same in terms of output results, although there may be some differences in the way you postprocess them or get the desired quantity. I suspect you’ve been confused or misinterpreted some of the results.
Second, are you aware that in the “waveguide with scatterer” example, we explicitly limit our calculation to Z-polarization only? However, we can easily change the settings and investigate all possible polarizations.
All in all, it makes sense to submit a request via our support portal with your model file, so that the reason for your confusion can be clarified and resolved.
Дмитрий Жабровец
October 14, 2024got it, sorry. I’ll study the models from the article more carefully
Ching-Ping Chang
March 27, 2025Hello,
You mentioned to set “Search for modes around” as the core refractive index. How about the “desired number of modes”?
How could this number be estimated? Or is this just a guessing game and find whichever number that make sense to the electric field distribution?
say if I am simulating a multimode optical fiber, would this just be the number I could estimated by the V number?
Thanks.
Sergey Yankin
April 1, 2025 COMSOL EmployeeHello! It is hard to give any specific instructions on how to estimate the Desired number of modes since this is pretty case-by-case. For a simple multimode fiber, I second your idea with the V-number usage. However, you should keep in mind that there might be degenerated modes for a circular waveguide within a solution. Let me put it differently, you might want to increase an estimation to compensate for it.
Garrett Beals
June 11, 2025Hi, thank you for the nice article. I am still confused about the axisymmetric case. I want to know what exactly is calculated when getting “emw.neff” and how “Rave” is calculated. I tried unsuccessfully to get Rave to output, but I could not find how the variable is stored. Thank you in advance!
Sergey Yankin
June 12, 2025 COMSOL EmployeeHi Garrett!
For a 2d axisymmetric case, as it is mentioned in the text, neff=-j*alpha/k0, with alpha=-lambda/Rave. Within the physics interface Rave is defined as PHYSICS.rAverage. Please keep in mind that you have an option to specify your own normalization if needed.
I encourage you to check the following tutorial out: https://www.comsol.com/model/step-index-fiber-bend-14189
Garrett Beals
June 12, 2025Hi Sergey, thanks for the quick reply. I have seen in some documentation that this average radius comes up in “advanced physics options” for COMSOL 6.X. However, I am using COMSOL 5.3 and am unable to find this option. Further, doing “emw.rAverage” does not give the average radius out. Can you please inform how to get average radius for COMSOL 5.3? Thanks!
I will also try to take a look at the tutorial as well.
Sergey Yankin
June 12, 2025 COMSOL EmployeeHello again, Garrett!
That automatic normalization on the physics level described in the blog post is a rather new addition to COMSOL, indeed. The approach for such an old version as 5.3 is a bit different. In fact, there is no normalization there, the output values are unscaled and there is no dedicated variable for the average radius.
To get the average radius in COMSOL 5.3, just do geometry-wise averaging for the r-coordinate.
For what it’s worth, I do encourage you to upgrade to the up-to-date COMSOL version since it has a lot of enhancements.
Ashish Rawat
August 5, 2025Hi, i am trying to obtain mode analysis for a fiber with variable core and cladding indices as per Sellmier equation for a range of wavlengths. The solution must bring out different values of effective index and core index for fundamental mode. However, i am getting both the values same for all wavelengths. Please assist me what possible reasons can be there which i need to address. (i already tried with PML and setting mode search near ncore_guess by defining it in parameters)
Sergey Yankin
August 6, 2025 COMSOL EmployeeHello Ashish! It’s hard to tell without seeing the model itself. The way you sweep the wavelength and do postprocessing must be checked. Please reach out to our support service with your model. Either my colleagues or I will be happy to help you.
Alex Dockery
August 11, 2025I am trying to model fiber optic cable with a core of one material with a coating of one with a slightly different refractive index. I got it all set up with the correct refractive numbers but I can not get a mode analysis to work. I would like to get all the Modes but even with it limited to 50 I just will not finish is this normal? What are the hardware requirement for something like this?
Sergey Yankin
August 11, 2025 COMSOL EmployeeHi Alex, it depends on the size to wavelength ratio. Do you happen to consider a multimode fiber with a big radius? This might be pretty demanding from the computational perspective. That said, this is a technical question, thus, I encourage you to reach out to our support service.
Joao Rebola
October 9, 2025Hello, when solving the mode analysis for a single-mode fiber, I get the two degenerate modes of the fundamental mode. I later want to extract data with them, but I am not sure about two things. 1) Comsol calculates its solution independently, so it does not split the power into the two degenerate modes? 2) Is there any way I can connect the tw degenerate modes? In my structure I have 2 cores. I get two solutions for each core independently, and in total, 4 combinations, when I need to do calculations. What is the right combination? Should I neglect some mode? Should I take into account the polarization of each mode?
Sergey Yankin
October 9, 2025 COMSOL EmployeeHi Joao!
I must admit that there are too many questions for an ordinary comment under the blog post, and not all of them are clear enough to give a comprehensive response. I encourage you to reach out to our support service.
A1: Well, you can technically call them independent solutions; however, I’m not sure I follow the purpose of this question.
A2: What is meant by the connection of two (?) modes? Why do you need it?
A3: What calculations do you need with respect to the 4 combinations mentioned? What’s the problem statement in the first place? Say, do you want to excite a particular mode in the subsequent frequency domain simulation? Or do you want to filter out some of the modes as irrelevant?
A4: It goes without saying that the polarization is of great importance regardless of the simulation. The counter-question is, again, what do you want to consider it for?